Redundancy and the Communication of Meaning in Music: Bach's 3-part Invention (Sinfonia) no. 9 in F minor

Hindemith chose Bach's F minor 3 part invention for analysis to demonstrate his theory of composition in "The craft of musical composition". It is a fascinating piece - one of Bach's most chromatic and expressive pieces of keyboard writing, and rather like other extraordinary musical moments (like Wagner's musical orgasm in Tristan), it raises the question "What is going on?". I like Hindemith's theory very much (although not as much as I like his music!), but his analysis sent me on my own analytical journey through the lens of information theory.

What happens in music, I believe, is the unfolding of a structure where multiple constraints are interwoven and overlaid. Information theory can provide some insight into this (as is discussed in a very recent paper from Loet Leydesdorff, myself and Inga Ivanova in the Journal of the Association for Information Science and Technology: https://onlinelibrary.wiley.com/doi/full/10.1002/asi.24052), and particularly the meaningfulness of the communication.

When considering music from the perspective of information theory, there are three fundamental problems to be overcome:

1. Music has no object of reference. So how is meaning communicated without reference?
2. Music emerges over time, producing novelty and unfolding a diachronic structure which appears to be linked to its synchronic structure. For this reason, music is not ergodic, unlike the use of letters in a language: its entropy over one period of time is not the same as its entropy over a different period of time. 
3. Music's unfolding novelty is not arbitrary: novelty in music appears to be a symmetry-breaking process similar to that found in epigenesis where both synchronic and diachronic symmetries gradually define structure

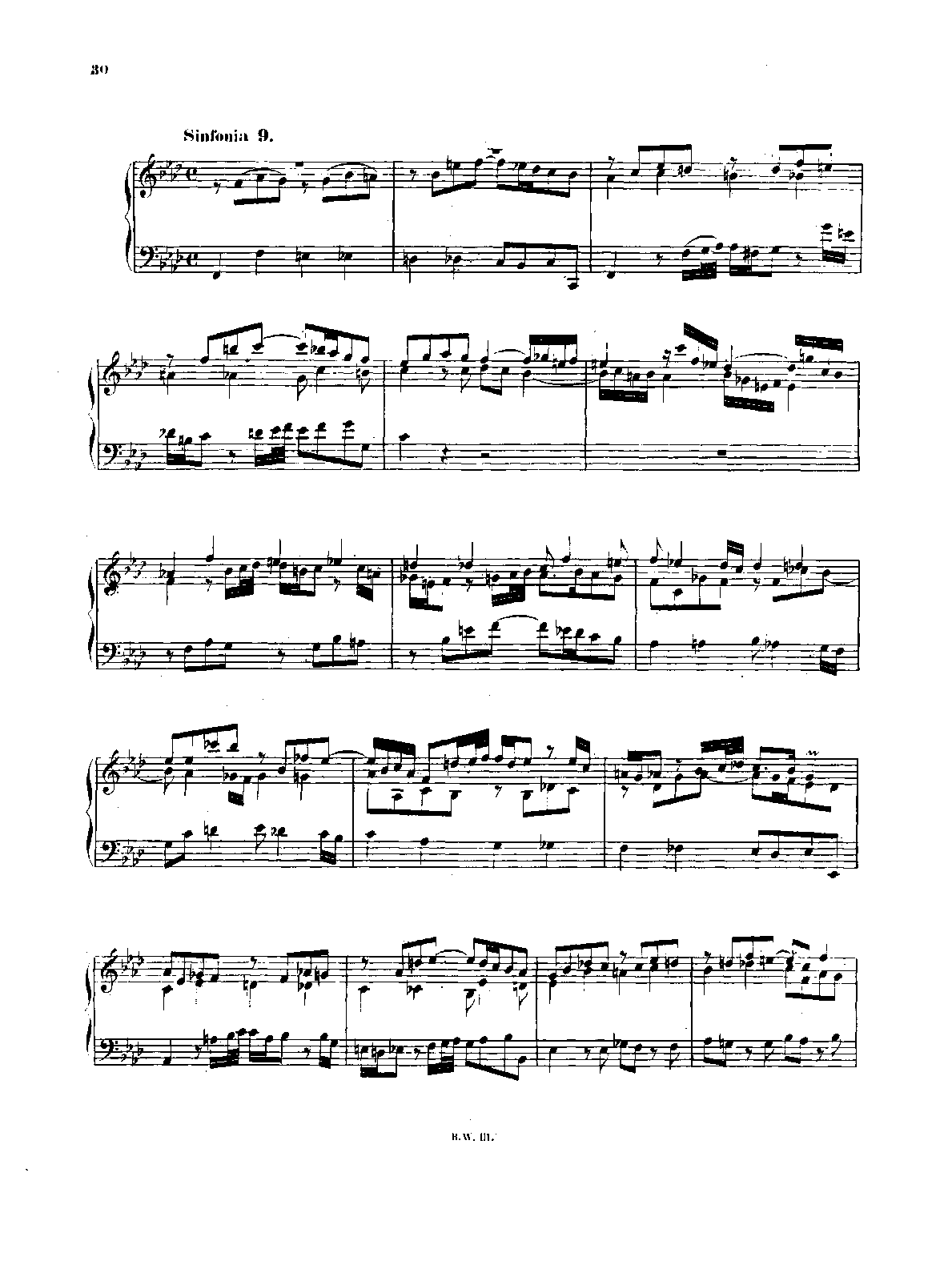
The first page of Bach's music looks like this:

Here's a performance:

The piece is fugal, and obviously in three parts, there is a very bare texture, and this bareness seems to contribute to the expressiveness of the music. However, there is a harmonic structure which is articulated throughout the piece, and a reduction of the harmonic written as chords per beat, looks something like this:

This kind of harmonic reduction is very common in music analysis as a method for getting at the "deep structure" of music (particularly in Schenker). It is typical of Bach's music that the harmonic reduction is very much like a chorale (hymn). In trying to understand how Bach's music works, we can start by asking about the relation between the harmonic reduction and the finished piece. 

At first glance, from an information theory perspective, the block chords of the reduction seem to remove a considerable amount of entropy which exists in the movement of parts in the original. It does this by compressing the variety into single "beats", which taken as an entirety have an entropy of 0. However, the variety compression makes more apparent the shifting harmonies. Written in chord symbols, this is an extended I (tonic) - V (dominant) - I (tonic) movement, interspersed with diminished chords (which are harmonically ambiguous) and a oscillation between major and minor chords. But if one was to calculate the entropy of the harmony, it wouldn't be that great. 

However, to say that the meaningfulness of Bach's music is arrived at by adding entropy is misleading. If entropy was to be added, we would expect increasing disorder. But if disorder is what is added, then it is unlikely that a coherent harmonic reduction would be possible, and furthermore it is unlikely that the piece would have any coherence. 

The striking thing about comparing the harmonic reduction with the original is that it is not disorder (entropy) which is added. It is redundancy.

This is most obvious is the melodic motif which Bach uses throughout the piece: 

This expresses a number of patterns which are represented throughout: the 3-quaver rhythm followed by a quaver pause; the interval jump of a third and then a fall of a second (except for moments of higher tension when he breaks this); and the phrasing with emphasis on the second note. 

In fugal writing, where each voice imitates others, the redundancy of patterns like this motif is enhanced. But it is interspersed with other ideas: the variety of the pattern is broken in the second bar, with a closing motif which also gets repeated throughout:

Shannon information theory, derived from Boltzmann's thermodynamics, measures uncertainty or disorder. It does this by identifying features which can be counted, and calculates the probabilities of their occurrences. 

On observing Bach's piece, the two motifs identified might be considered to be "features", and their occurrences over time calculated. Equally, the occurrences of individual notes might be calculated, or the harmonic sequence identified in the harmonic reduction. But somehow this seems to miss the point. When we do Shannon analysis in text, we can identify words, and usually those words have reference, and so the communication of meaning can be inferred from the patterns of the words and their references (this is how big data techniques like Topic Modelling work)

The second problem about music's lack of ergodicity is more serious still. Whatever is counted at the beginning is not what can be counted at the end. Novelty arises through the unfolding. Since Shannon's formulae requires an index of features, how might it accommodate features which cannot be foreseen from the outset?

However, there are fundamental features for which the entropies can be calculated: notes, rhythm, intervals, harmony, etc. An emergent structure can be arrived at through the interaction between different constraints: music creates its own constraints. Every measurement of entropy of a particular feature is constrained by the measurements of entropies of other features: the choice of notes depends on the choice of harmony or rhythm, for example.

Seeing any measurement of entropy in this way means that any measurement of entropy is also an index of the constraints (or redundancies) that produce that measure of entropy. In this way, we do not need to engage with Shannon's formula for redundancy - which is good because it invokes the notion of "maximum entropy" which is a concept that is beyond measurement. 

Constraints work over time. The effect of one constraint on another results in changes to the entropies of different features. If one constraint changes at the same time as another, then there is some connection between them.

In considering the motif that Bach uses to structure his fugue, the intervals, the rhythm, the expression and the pattern of notes are all the same. That means that the entropies of each fundamental element relative to the others is tightly coupled. 

Does this means that tight coupling between fundamental features is a sign of an emergent cell or motif? I think it might, and of course, if tight coupling is identified as a new idea, then the new idea becomes a new feature in the analysis, upon which its own entropy can be calculated relative to other features. 

At the end of a piece, there is tight coupling between everything. Everything dissolves into silence. The important thing is that everything dissolves into what it came from. This is directly related to problem 3 above: novelty is not arbitrary. All novelty articulates different descriptions of the whole. Nothing (silence) is arrived at when those descriptions come together in the same way that the colour spectrum comes together to produce white light. 

Music is profound in its communication of meaning because it expresses something fundamental in our own biological structure. In music's unfolding broken symmetry, we recognise our own unfolding broken symmetry. This principle is behind what I think Alfred Schutz saw in his paper "Making Music Together". When education really works, it has exactly this same property expressed in the loving relations between human beings.